Integrate Functions Using Subtitution

 

Substitution could be used to find the antiderivative of an integrated function easier. It is also called u-substitution. Substitution is commonly used when we could not take the integral of a function using ordinary, common rules, such as power rule or trigonometric rules. It is to find a way to reverse the chain rule and make it facile for us to find the antiderivative using the rules that we know. This technique could be used in both definite and indefinite integrals. 

 

Using u-substitution for Indefinite Integrals 

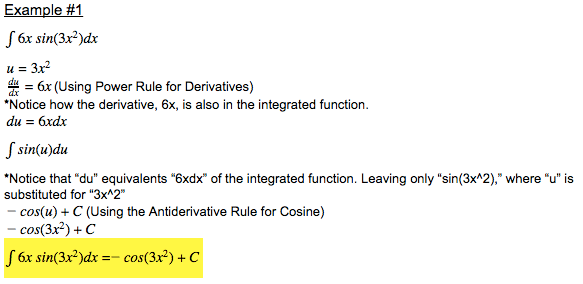
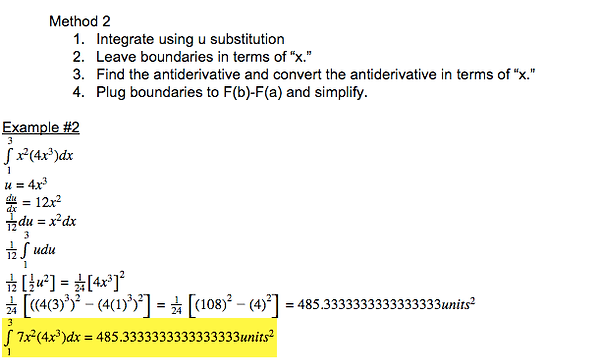
Find the “u” of the integrated function. The “u” is most often the function that contains the “x,” the function that is inside the parenthesis (the inside function), the function that have the highest power, or the function that is in the denominator.

 

• It is all to try to make the derivative of “u” the same as a coefficient or a function that is present in the original function.

• If not, we could also manipulate “u” to look like a version of some function or coefficient in the original function.  

 

Take the derivative of the “u.” 

 

If the derivative of “u” is the same as a function or coefficient in the original function, attach “dx” to the other side to isolate “du.” If the derivative is similar (not the same), move the coefficient of the derivative to “du” and move “dx” to the other side so “du” will be the same as a function or a coefficient in the original function.  

 

Plug “u” and “du” back into the original function to replace “x” and “dx.” If there is a coefficient inside the integral, move the coefficient in front of the integral via Scalar Multiplication with Integrals rule. 

 

Take the integral of the function and add “C” to the antiderivative to represent a possible constant. 

 

Plug the function that represents “u” to replace “u.”