
Global/Local Minimas and Maximas
Critical Point: The peaks (high points) and valleys (low points) of a function.
Critical Values: The outputs (y-value) of critical points.
Inflection Points: The point on the graph where f”(x) changes concavity (changes signs). Inflection points in f”(x) are either zero or undefined.
Local Maximum: A function have a local maximum at p if f(p) is greater than or equal to the other values that are near p.
Local Minimum: A function have a local minimum at p if f(p) is less than or equal to the other values that are near p.
Global Maximum: A single greatest value over the entire function.
(If the graphed function are arrowed, then the global maximum is undefined)
Global Minimum:A single lowest value over the entire function.
(If the graphed function are arrowed, then the global minimum is undefined).
Inflection Points and Local Maxima and Minima of the Derivative: A continuous function that has an inflection point at p if f’ has a local maximum or local minimum at p and if f” changes signs at p.
First Derivative Test for Local Maxima and Minima
If f’(x) changes from positive to negative at critical point, then f(x) has a local max at that critical point.
If f’(x) changes from negative to positive at critical point, then f(x) has a local min at that critical point.
If f’(x) has a critical point, but the derivative doesn’t change signs at the critical point, then there is no local max or local min at that critical point.
Second Derivative Test for Local Maxima and Minima
Find critical point first.
If f”(x) is positive at the critical point, then f(x) has a local min at that critical point.
If f”(x) is negative at critical point, then f(x) has a local max at that critical point.
Calculating Maxima and Minimina
For the function, , find all critical points, local maxima, local minima, inflection points, global maxima, and global minima over interval [-5, 10].
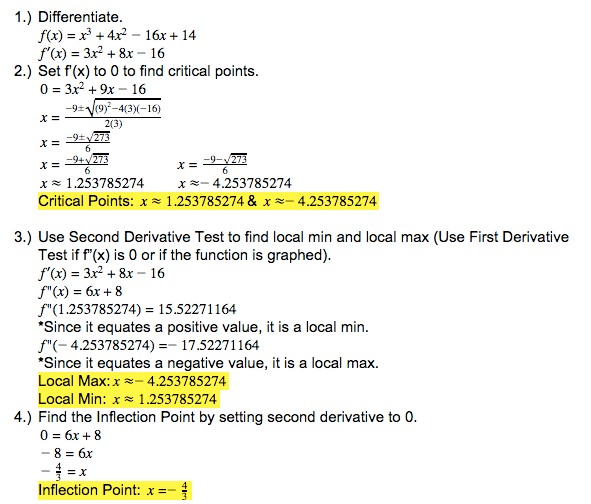

Inflection Points
If f' has a local max or local min at p and f" changes signs at p, then f has an inflection point at p.
Global Maxima and Global Minima
-
To find the global min and max of a continuous function ona closed interval, compare the values of the function at all of the critical points and at the endpoints.
-
To find the global max and min on an open interval, find the value of the function at all critical points and sketch or examine the graph of the function. Focus on the function values as x approaches positive and negative infinity.